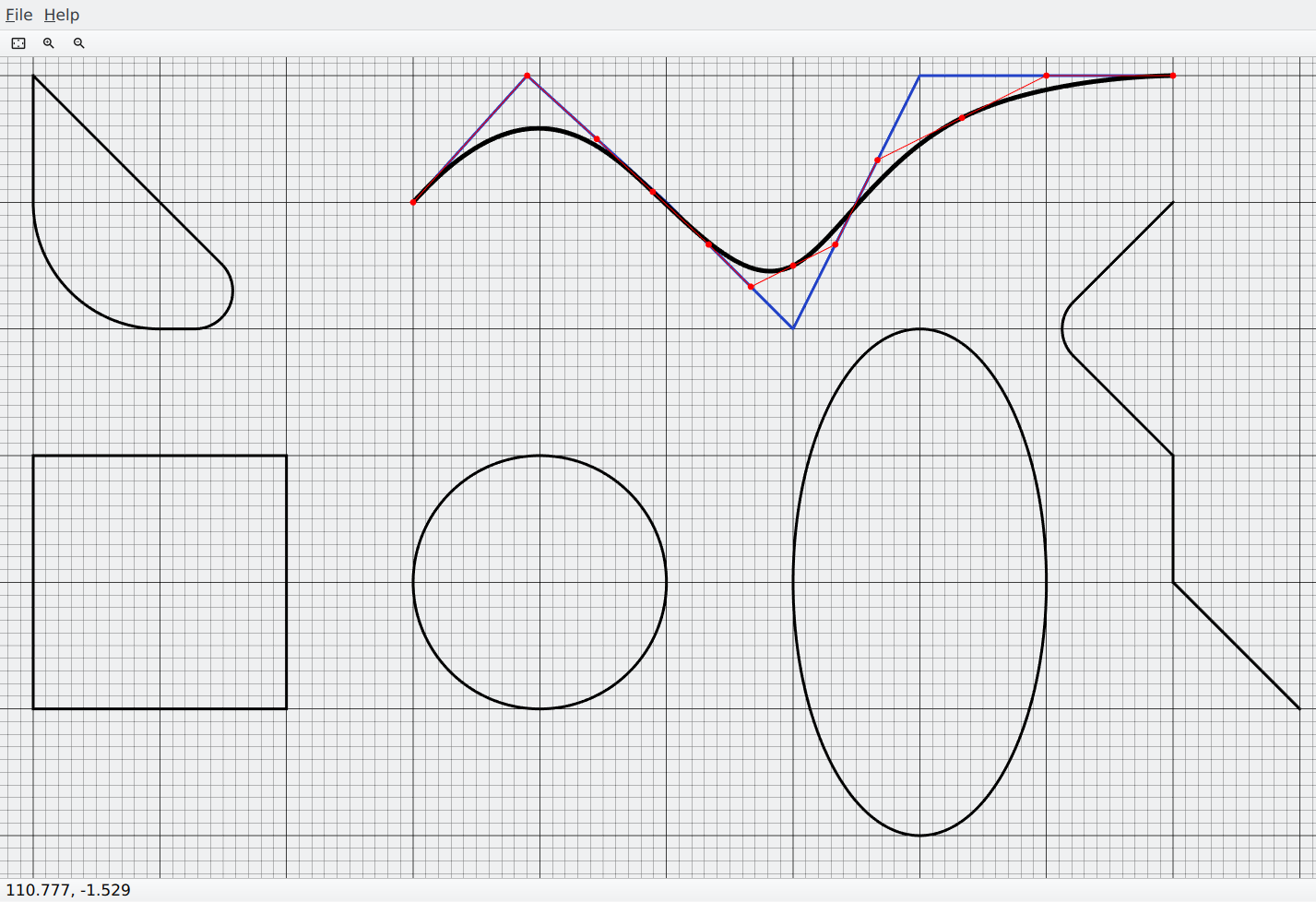
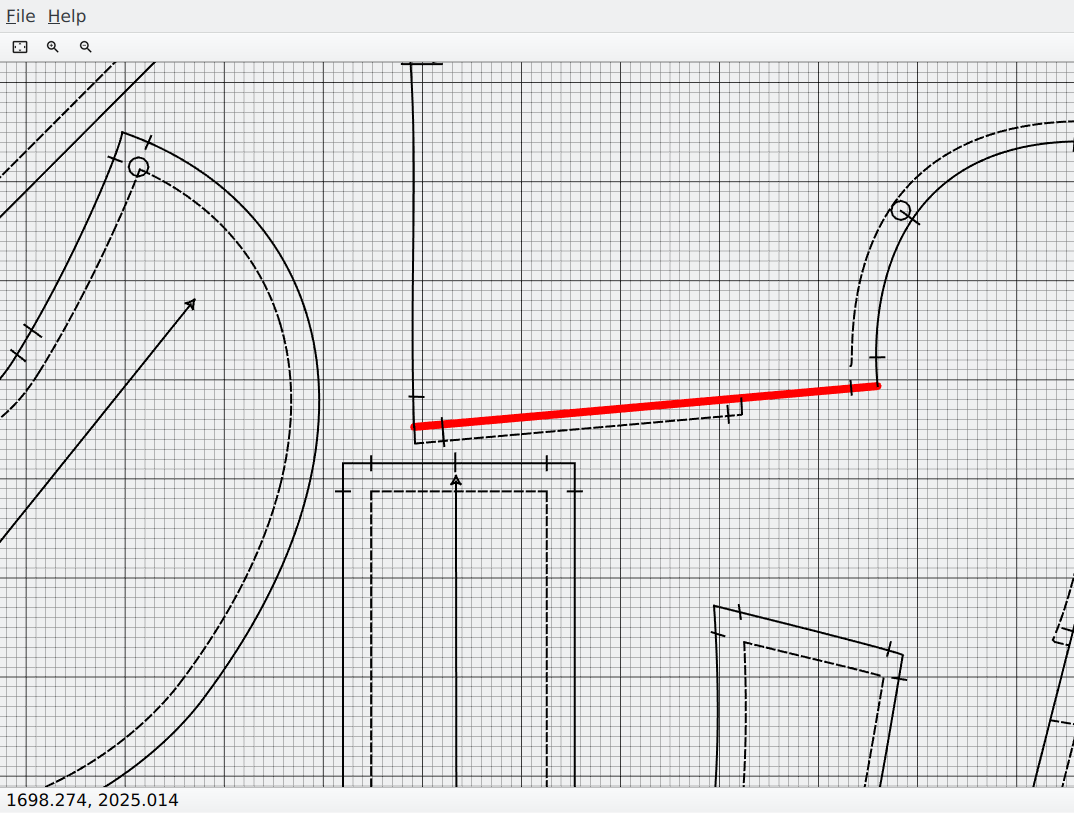
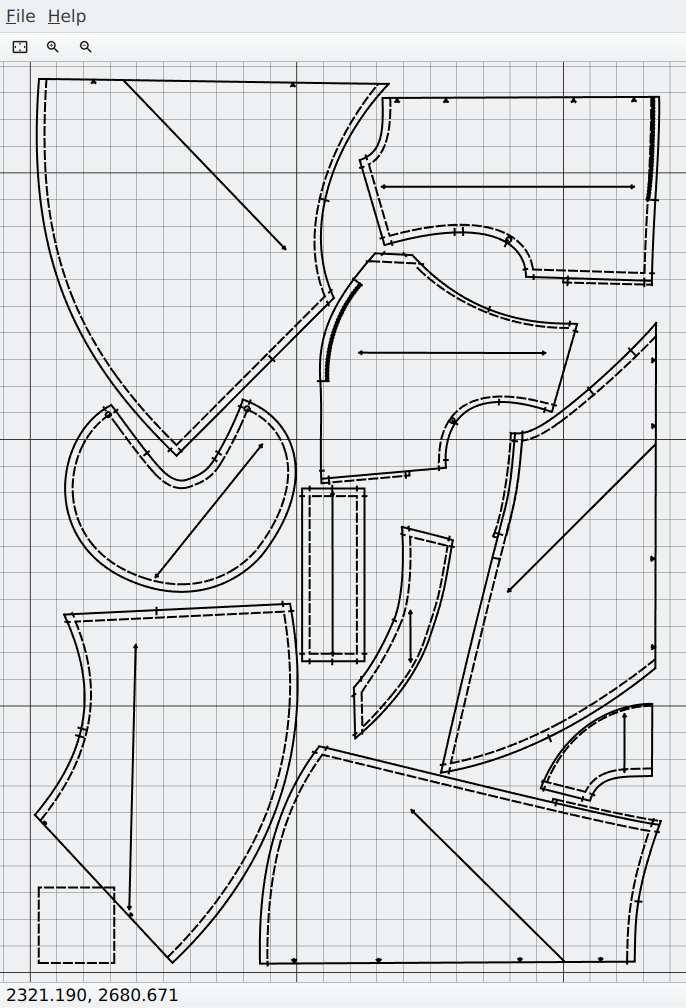
61.5 KiB
60.3 KiB
72.8 KiB
doc/sphinx/source/design-notes/index.rst
0 → 100644
doc/sphinx/source/examples/dxf-import.rst
0 → 100644
doc/sphinx/source/examples/svg-import.rst
0 → 100644
doc/sphinx/source/graphic-engine-review.rst
0 → 100644